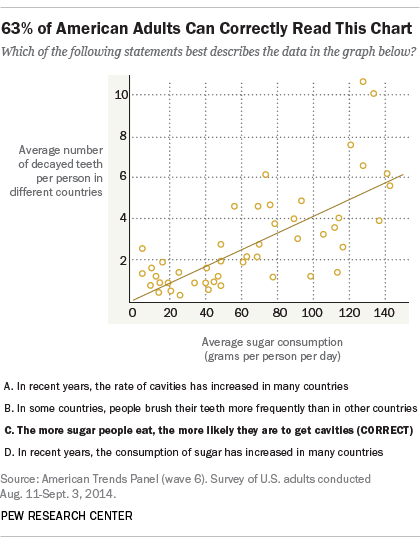
Scatter graph method
She reviews department records to determine the activity levels for those same months. After gathering data from these two places, the accountant has all the information she needs to perform the analysis. Thus, the scatter diagram method is the simplest device to study the degree of relationship between the variables by plotting the dots for each pair of variable values given. The chart on which the dots are plotted is also called as a Dotogram.
A correlation of -0.97 is a strong negative correlation while a correlation of 0.10 would be a weak positive correlation. A low Pearson correlation coefficient does not mean that no relationship exists between the variables. To check for nonlinear relationships graphically, create a scatterplot or use simple regression. One method of estimating mixed costs is by preparing a scatter graph.
Remember, correlation strength is measured from -1.00 to +1.00. The correlation coefficient often expressed as r, indicates a measure of the direction and strength of a relationship between two variables. When the r value is closer to +1 or -1, it indicates that there is a stronger linear relationship between the two variables.
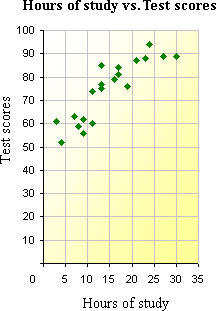
Scatter plot
You can reduce this potential problem by collecting information at other activity levels and affirming the fixed and variable relationships at these other levels. The result could be that the furthest data points are thrown out, resulting in a more reliable high-low analysis. Where the correlation coefficient is 0 this indicates there is no relationship between the variables (one variable can remain constant while the other increases or decreases).
What are the 3 types of scatter plots?
A scattergraph has a horizontal x-axis that represents production activity, a vertical y-axis that represents cost, data that are plotted as points on the graph, and a regression line that runs through the dots to represent the relationship between the variables.
A regression line can be used to statistically describe the trend of the points in the scatter plot to help tie the data back to a theoretical ideal. This regression line expresses a mathematical relationship between the independent and dependent variable. Depending on the software used to generate the regression line, you may also be given a constant that expresses the ‘goodness of fit’ of the curve. That is to say, to what degree of certainty can we say this line truly describes the trend in the data. The correlational constant is usually expressed as R2 (R-squared).
In the case that there are a few outliers (data points that are located far away from the rest of the data) the line will adjust so that it represents those points as well. The scatter diagram graphs pairs of numerical data, with one variable on each axis, to look for a relationship between them.
Understanding the Scattergraph Method
If the correlation coefficient has a negative value (below 0) it indicates a negative relationship between the variables. This means that the variables move in opposite directions (ie when one increases the other decreases, or when one decreases the other increases).
For example, weight and height, weight would be on y axis and height would be on the x axis. Correlations may be positive (rising), negative (falling), or null (uncorrelated). If the pattern of dots slopes from lower left to upper right, it indicates a positive correlation between the variables being studied.
The concept is useful in the analysis of pricing and the derivation of budgets. It could be used to determine the fixed and variable components of the costs associated with a product, product line, machine, store, geographic sales region, subsidiary, or customer.
A value of zero indicates that there is no relationship between the two variables. The scatter graph method is used to segregate mixed costs and is more accurate than the high-low mehod. By plotting relevant data points in a graph, the fixed and variable cost components can be determined. The high-low method is used to discern the fixed and variable portions of a mixed cost. The essential concept is to collect the cost at a high activity level and again at a low activity level, and then extract the fixed and variable cost components from this information.
Scatter plot matrices
- The scattergraph method is a visual technique for separating the fixed and variable elements of a semi-variable expense (also called a mixed expense) in order to estimate and budget for future costs.
- A scatter plot can suggest various kinds of correlations between variables with a certain confidence interval.
- For example, weight and height, weight would be on y axis and height would be on the x axis.
For each month, you need the total of the cost and the total of the activity. The activity for utilities could be kilowatts used, gallons used, hours used, etc. Plot on the scattergraph a regression line that represents the relationship between the various data points.
Another advantage of this method is that it only requires two sets of numbers to calculate the fixed and variable costs. The accountant reviews the financial transactions for the account over several months to obtain the total cost amount.
Anytime the correlation coefficient, denoted as r, is greater than zero, it’s a positive relationship. Conversely, anytime the value is less than zero, it’s a negative relationship.
The following plots show data with specific Spearman correlation coefficient values to illustrate different patterns in the strength and direction of the relationships between variables. The larger the absolute value of the coefficient, the stronger the relationship between the variables. A line of best fit is often useful to attempt to represent data with the equation of a straight line in order to predict values that may not be displayed on the plot. The line of best fit is determined by the correlation between the two variables on a scatter plot.
The accountant lists each set of data and identifies the high and low values. She divides the difference in dollars by the difference in activity to calculate the cost per unit of activity, or the variable activity. She multiplies the variable cost per unit by the number of activities to calculate the total variable cost. She subtracts the total variable cost from the total cost to determine the fixed cost. In cost accounting, the high-low method is a way of attempting to separate out fixed and variable costs given a limited amount of data.
If the pattern of dots slopes from upper left to lower right, it indicates a negative correlation. A line of best fit (alternatively called ‘trendline’) can be drawn in order to study the relationship between the variables. An equation for the correlation between the variables can be determined by established best-fit procedures.
A scatter plot is also very useful when we wish to see how two comparable data sets agree to show nonlinear relationships between variables. The ability to do this can be enhanced by adding a smooth line such as LOESS. Furthermore, if the data are represented by a mixture model of simple relationships, these relationships will be visually evident as superimposed patterns. Another disadvantage of the high-low method is the number of steps necessary to perform this analysis. The accountant needs to gather monthly data regarding the expense being analyzed and the unit of activity.
The total amount of fixed costs is assumed to be the same at both points of activity. The change in the total costs is thus the variable cost rate times the change in the number of units of activity. The costs associated with a product, product line, equipment, store, geographic sales region, or subsidiary, consist of both variable costs and fixed costs. To determine both cost components of the total cost, an analyst or accountant can use a technique known as the high-low method.
Whether this regression line should be linear or curved depends on what your hypothesis predicts the relationship is. When a curved line is used, it is typically expressed as either a second order (cubic) or third order (quadratic) curve. Higher order curves may follow the actual data points more closely, but rarely provide a better mathematical description of the relationship. The high-low method is used to calculate the variable and fixed cost of a product or entity with mixed costs. It considers the total dollars of the mixed costs at the highest volume of activity and the total dollars of the mixed costs at the lowest volume of activity.
A scatter graph splits the cost into fixed and variable portions. Creating a scatter graph can help you determine the future amounts of a cost. The data from a completed scatter graph provides the information you need to create a cost equation for each of your mixed costs. The cost equation will provide you with fixed and variable estimates for each mixed cost. You will need historical data to create the scatter graph; one year’s worth is the minimum to get a good approximation of the expense.
Using Common Stock Probability Distribution Methods
For a linear correlation, the best-fit procedure is known as linear regression and is guaranteed to generate a correct solution in a finite time. No universal best-fit procedure is guaranteed to generate a correct solution for arbitrary relationships.
A typical regression line has an upward slant, indicating that costs increase with unit volume. The regression line may also intercept the y axis above the zero cost level, indicating the presence of fixed costs that must be incurred even in the absence of any unit activity.
The high-low method involves taking the highest level of activity and the lowest level of activity and comparing the total costs at each level. The range of values for the correlation coefficient is -1.0 to 1.0. In other words, the values cannot exceed 1.0 or be less than -1.0 whereby a correlation of -1.0 indicates a perfectnegative correlation, and a correlation of 1.0 indicates a perfectpositive correlation.
The scattergraph method is a visual technique for separating the fixed and variable elements of a semi-variable expense (also called a mixed expense) in order to estimate and budget for future costs. A scatter plot can suggest various kinds of correlations between variables with a certain confidence interval.
If the variables are correlated, the points will fall along a line or curve. The better the correlation, the tighter the points will hug the line. This cause analysis tool is considered one of the seven basic quality tools. Because the data points represent real data collected in a laboratory setting rather than theoretically calculated values, they will represent all of the error inherent in such a collection process.